Class 10 RD Sharma Solutions – Chapter 14 Coordinate Geometry – Exercise 14.2 | Set 2

Last Updated : 01 Nov, 2022

Question 20. The three vertices of a parallelogram are (3, 4), (3, 8), and (9, 8). Find the fourth vertex.

Solution:

Let ABCD be a parallelogram and vertices will be A (3, 4), B (3, 8), C (9, 8)

and the co-ordinates of fourth vertex D be(x, y)

Now AB = 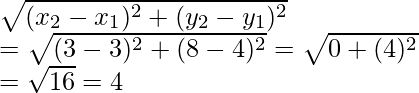$\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ =\sqrt{(3-3)^2+(8-4)^2}=\sqrt{0+(4)^2}\\ =\sqrt{16}=4$

Similarly, BC = 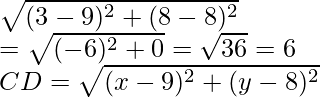$\sqrt{(3-9)^2+(8-8)^2}\\ =\sqrt{(-6)^2+0}=\sqrt{36}=6\\ CD=\sqrt{(x-9)^2+(y-8)^2}$

and DA = 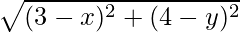$\sqrt{(3-x)^2+(4-y)^2}$

Hence, ABCD is a ||gm

Here, AB = CD and BC = AD

CD = 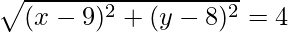$\sqrt{(x-9)^2+(y-8)^2}=4$

On squaring both sides we get

(x – 9)²+ (y – 8)²= (4)²

x²– 18x + 81 + y²– 16y + 64 = 16

x²+ y²– 18x – 16y = 16 – 81 – 64

x²+ y²– 18x – 16y = -129 ………..(i)

Similarly, AD = 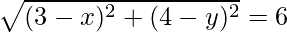$\sqrt{(3-x)^2+(4-y)^2}=6$

On squaring both sides we get

(3 – x)²+ (4 – y)²= 26

9 + x²– 6x + 16 + y²– 8y = 36

x²+ y²– 6x – 8y = 36 – 9 – 16

x²+ y²– 6x – 8y = 11 ………..(ii)

Now on subtracting eq(i) from (ii), we get

12x + 8y = 140

3x + 2y = 35

2y = 35 – 3x

y = (35 – 3x)/2 ………..(iii)

Now substituting the value of y in eq (ii)

$x^2(\frac{35-3x}{2})^2-6x-8(\frac{35-3x}{2})=11\\ x+\frac{1225+9x^2-210x}{4}-6x-140+12x=11$

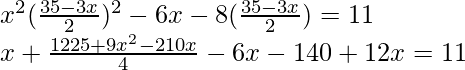
4x²+ 1225 + 9x²– 210x – 24x – 560 = 44

13x²– 186 + 621 = 0

13x²– 117x – 69x + 621 = 0

13x(x – 9) – 69(x – 9) = 0

(x – 9)(13x – 69) = 0

Either x – 9 = 0, then x = 9

or 13x – 69 = 0, then x69/13 which is not possible

So, x = 9

Hence, the vertex will be (9,4)

Question 21. Find a point which is equidistant from the point A (-5, 4) and B (-1, 6). How many such points are there?

Solution:

Let us considered P (h, k) be the point which is equidistant from the points A (-5, 4) and B (-1, 6).

So, PA = PB

Therefore, (PA)² = (PB)²

Now by distance formula, we get

(-5 – h)²+ (4 – k)²= (-1 – h)²+ (6 – k)²

25 + h²+ 10h + 16 + k²– 8k = 1h²+ 2h + 36 + k²– 12k

25 + 10h + 16 – 8k = 1 + 2h + 36 – 12k

8h + 4k + 41 – 37 = 0

8h + 4k + 4 = 0

2h + k + 1 = 0 …….(i)

Midpoint of AB = ((-5 – 1)/2, (4 + 6)/2) = (-3, 5)

At point (-3, 5), from eq(i)

2h + k = 2(-3) + 6

-6 + 5 = -1

2h + k + 1 = 0

So, the mid-point of AB satisfy the Eq. (i).

Hence, infinite number of points, in fact all points which are solution

of the equation 2h + k + 1 = 0, are equidistant from the point A and B.

Replacing h, k, by x, y in above equation, we have 2x + y + 1 = 0

Question 22. The centre of a circle is (2a, a – 7). Find the values of a if the circle passes through the point (11, -9) and has diameter 10√2 units.

Solution:

According to the question

Distance between the centre C (2a, a – 1) and the point P (11, -9), which lie on the circle = Radius of circle

So, Radius of circle = $\sqrt{(11-2a)^2+(-9-a+7)^2}$ …..(i)

Given that, length of diameter = 10√2

Therefore, length of radius = Length of diameter/2

= 10√2/2 = 5√2

Now put this value in eq(i), we get

$5\sqrt{2}=\sqrt{(11-2a)^2+(-2-a)^2}$

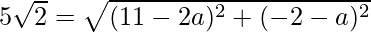
Squaring on both sides, we get

50 = (11 – 2a)²+ (2 + a)²

50 = 121 + 4a² – 44a + 4 + a²+ 4a

5a²– 40a + 75 = 0

a²– 8a + 15 = 0

a²– 5a – 3a + 15 = 0

a(a – 5) – 3(a – 5) = 0

(a – 5)(a – 3) = 0

So, a = 3, 5

Hence, the required values of a are 5 and 3.

Question 23. Ayush starts walking from his house to office. Instead of going to the office directly, he goes to a bank first, from there to his daughter’s school and then reaches the office. What is the extra distance travelled by Ayush in reaching the office? (Assume that all distance covered are in straight lines). If the house is situated at (2, 4), bank at (5, 8), school at (13, 14), and office at (13, 26), and coordinates are in kilometers.

Solution:

According to the figure

Distance between two points $d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}$

Now, distance between house and bank

$=\sqrt{(5-2)^2+(8-4)^2}\\ =5$

Distance between bank and daughter’s

School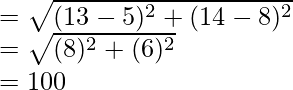 $=\sqrt{(13-5)^2+(14-8)^2}\\ =\sqrt{(8)^2+(6)^2}\\ =100$

Distance between daughter’s school and office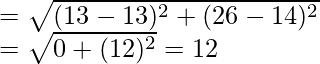 $=\sqrt{(13-13)^2+(26-14)^2}\\ =\sqrt{0+(12)^2}=12$

Total distance (house+bank+school+office) travelled = 5 + 10 + 12 = 27 units

Distance between house to offices

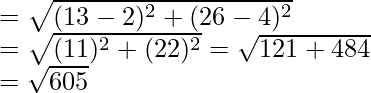$=\sqrt{(13-2)^2+(26-4)^2}\\ =\sqrt{(11)^2+(22)^2}=\sqrt{121+484}\\ =\sqrt{605}$ = 24.59 = 24.6km

So, the extra distance travelled by Ayush in reaching his office = 27 – 24.6 = 2.4km.

Hence, the required extra distance travelled by Ayush is 2.4 km

Question 24. Find the value of k, if the point P (0, 2) is equidistant from (3, k) and (k, 5).

Solution:

Let us considered P (0, 2) is equidistant from A (3, k) and B (k, 5)

PA = PB

=> PA² = PB²

$=\sqrt{(0-3)^2+(2-k)^2}$

Therefore, PA²= (0 – 3)²+ (2 – k)²

= (-3)²+ (2 – k)²

= k²– 4k + 13

Similarly, PB²= (k – 0)²+ (5 – 2)²

= k²+ (3)²= k²+ 9

Therefore, PA = PB

PA²= PB²

k²– 4k + 13 = k²+ 9

-4k = 9 – 13

-4k = -4

k = -4/-4 = 1

Hence, K = 1.

Question 25. If (-4, 3) and (4, 3) are two vertices of an equilateral triangle, find the coordinates of the third vertex, given that the origin lies in the

(i) interior,

(ii) the exterior of the triangle.

Solution:

Let us considered the third vertex of an equilateral triangle be (x, y).

So, the vertices are A (-4, 3), B (4,3) and C (x, y).

We know that, in equilateral triangle the angle between two

adjacent side is 60 and all three sides are equal.

Therefore, AB = BC = CA

AB²= BC²= CA² …..(i)

Now, taking first two parts

AB²= BC²

(4 + 4)²+ (3 – 3)²= (x – 4)²+ (y – 3)²

64 + 0 = x²+ 16 – 8x + y²+ 9 – 6y

x²+ y²– 8x – 6y = 39 …..(ii)

Now, taking first and third parts.

AB²= CA²

(4 + 4)²+ (3 – 3)²= (x – 4)²+ (y – 3)²

64 + 0 = x²+ 16 – 8x + y²+ 9 – 6y

x²+ y²– 8x – 6y = 39

On subtracting eq(ii) from (iii), we get

x = 0

Now, put the value of x in eq(ii), we get

0 + y²– 0 – 6y = 39

y²– 6y – 39 = 0

Therefore, y = $\frac{6±\sqrt{(-6)^2-4(1)(-39)}}{2*1}$

$y=\frac{6±\sqrt{36+156}}{2}\\ y=\frac{6±\sqrt{192}}{2}\\ y=\frac{6±2\sqrt{48}}{2}=3±\sqrt{48}\\ y=3±4\sqrt{3}\\ y=3+4\sqrt{3}or\space 3-4\sqrt{3}$

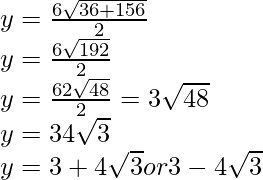
So, the points of third vertex are

(0, 3+√3)or(3 – 4√3)

But it is given that, the origin lies in the interior of the ∆ABC and the x-coordinate of third vertex is zero.

So, y-coordinate of third vertex should be negative.

Hence, the requirement coordinate of third vertex,

C = (0, 3 – 4√3)

Question 26. Show that the points (-3, 2), (-5, -5), (2, -3), and (4, 4) are the vertices of a rhombus. Find the area of this rhombus.

Solution:

Let us considered the co-ordinates of the vertices A, B, C and D of a rhombus are A (-3, 2), B (-5, -5), C (2, -3) and D (4, 4)

$AB=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ \sqrt{(-5+3)^2+(-5-2)^2}=\sqrt{(-2)^2+(-7)^2}$

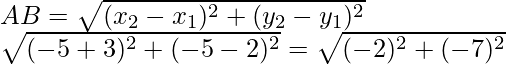
or AB²= (-2)²+ (-7)²= 4 + 49 = 53

Similarly, BC²= (2 + 5)²+ (-3 + 5)²

= (7)²+ (2)²= 49 + 4 = 53

CD²= (4 – 2)²+ (4 + 3)²

= (2)²+ (7)²= 4 + 48 = 53

and DA²= (-3 – 4)²+ (2 – 4)²

= (-7)²+ (-2)²= 49 + 4 = 53

Therefore, we see that AB = BC = CD = DA = √53

Now diagonals AC = $\sqrt{(2+3)^2+(-3-2)^2}\\ =\sqrt{(5)^2+(-5)^2}=\sqrt{25+25}\\ =\sqrt{50}=\sqrt{25*2}=5\sqrt{2}\\$

and diagonal BD = $\sqrt{(4+5)^2+(4+5)^2}\\ =\sqrt{(9)^2+(9)^2}=\sqrt{81+81}=\sqrt{162}\\ =\sqrt{81*2}=9\sqrt{2}$

Therefore, sides are equal but diagonal are not equal

Hence, ABCD is a rhombus

Now we find the area of rhombus = Product of diagonal/2

= (5√2 × 9√2)/2

= 9/2

= 45sq.units

Question 27. Find the coordinates of the circumcenter of the triangle whose vertices are (3, 0), (-1, -6), and (4, -1). Also, find its circumradius.

Solution:

Let us considered ABC is a triangle whose vertices are A (3, 0), B (-1, -6) and C (4, -1)

So, O be the circumcenter of the triangle ABC and so the co-ordinates be (x, y)

Therefore, OA = OB = OC

OA²= OB²= OC²

Now OA = 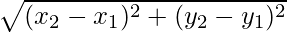$\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\$

OA²= (x₂– x₁)²+ (y₂– y₁)²

= (x – 3)²+ (y – 0)²

= (x – 3)²+ y²

OB²= (x + 1)²+(y + 6)²

and OC²= (x – 4)²+ (y + 1)²

Therefore, OA²= OB²

(x – 3)²+ y²= (x + 1)²+ (y + 6)²

x²– 6x + 9 + y²= x²+2x + 1 + y²+ 12y + 36

-6x – 2x – 12y = 1 + 36 – 9

-8x – 12y = 28

2x + 3y = -7 ……..(i)

Therefore, OB²= OC²

(x + 1)²+ (y + 6)²= (x – 4)²+ (y + 1)²

x²+ 2x + 1 + y²+ 12y + 36 = x²– 8x + 16 + y²+ 2y + 1

2x + 12y + 37 + 8x – 2y = 17

10x + 10y = 17 – 37 = -20

x + y = -2 ……..(ii)

On multiply eq(i) by 1 and (ii) by 2, we get

2x + 3y = -7

2x + 2y = -4

Now, on substituting y = -3, we get

x + y = -2

x – 3 = -2

x = -2 + 3 = 1

Radius = OA = 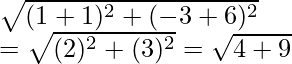$\sqrt{(1+1)^2+(-3+6)^2}\\ =\sqrt{(2)^2+(3)^2}=\sqrt{4+9}$ = √13

Question 28. Find a point on the x-axis which is equidistant from the points (7, 6) and (-3, 4).

Solution:

The required point is on x-axis

Its ordinate will be O

So, the co-ordinates of the required point P (x, 0)

It is given that the point P is equidistant from the points A (7, 6) and B (-3, 4)

Now AP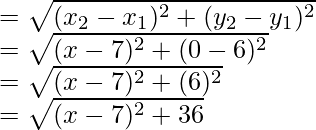 $=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ =\sqrt{(x-7)^2+(0-6)^2}\\ =\sqrt{(x-7)^2+(6)^2}\\ =\sqrt{(x-7)^2+36}$

Therefore, AP²= (x – 7)²+ 36

Similarly, BP²= (x + 3)²+ (0 – 4)²

= (x + 3)²+ 16

Therefore, AP = BP

AP²= BP²

Therefore, (x – 7)²+ 36 = (x + 3)²+ 16

x²– 14x + 49 + 36 = x²+ 6x + 9 + 16

x²– 14x – x²– 6x = 25 – 85

-20x = -60

x = -60/-20 = 3

Therefore, the required point will be (3, 0)

Question 29. (i) Show that the points A (5, 6), B (1, 5), C (2, 1), and D (6, 2) are the vertices of a square.

(ii) Prove that the points A (2, 3), B (-2, 2), C (-1, -2), and D (3, -1) are the vertices of a square ABCD.

(iii) Name the type of triangle PQR formed by the point P(√2, √2), Q(- √2, – √2), and R (-√6, √6).

Solution:

(i) Given points are given A (5, 6), B (1, 5), C (2, 1) and D (6, 2)

Now AB²= (x₂– x₁)²+ (y₂– y₁)²

= (1 – 5)²+ (5 – 6)²

= (-4)²+ (-1)²= 16 + 1 = 17

Similarly, BC = (2 – 1)²+ (1 – 5)²

= (1)²+ (-4)²

= 1 + 16 = 17

CD = (6 – 2)²+ (2 – 1)²+ (4)²+ (1)²

= 16 + 1 = 17

and DA = (5 – 6)²+ (6 – 2)²

= (-1)²+ (4)²

= 1 + 16 = 17

Diagonals AC²= (2 – 5)²+ (1 – 6)²

= (-3)²+ (-5)²

= 9 + 25

=34

and BD²= (6 – 1)²+ (2 – 5)²

= (5)²+ (-3)²

= 25 + 9 = 34

We conclude that

AB = BC = CD = DA and diagonals AC = BD

Hence, ABCD is a square.

(ii) Given points A(2, 3), B(-2, 2), C(-1, -2) and D(3, -1)

$AB=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ =\sqrt{()^2+(2-3)^2}\\ =\sqrt{(-4)^2+(-1)^2}\\ =\sqrt{16+1}=\sqrt{17}$

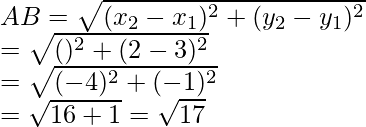
Similarly,

$BC=\sqrt{(-1+2)^2+(-2-2)^2}\\ =\sqrt{(1)^2+(-4)^2}\\ =\sqrt{1+16}=\sqrt{17}\\ CD=\sqrt{(3+1)^2+(-1+2)^2}\\ =\sqrt{(4)^2+(1)^2}\\ =\sqrt{16+1}=\sqrt{17}\\ DA=\sqrt{(2-3)^2+(3+1)^2}\\ =\sqrt{(-1)^2+(4)^2}\\ =\sqrt{1+16}=\sqrt{17}\\ AC=\sqrt{(-1-2)^2+(-2-3)^2}\\ =\sqrt{(-3)^2+(-5)^2}\\ =\sqrt{9+25}=\sqrt{34} \\ BD=\sqrt{(3+2)^2+(-1-2)^2}\\ =\sqrt{(5)^2+(-3)^2}\\ =\sqrt{25+9}=\sqrt{34}$

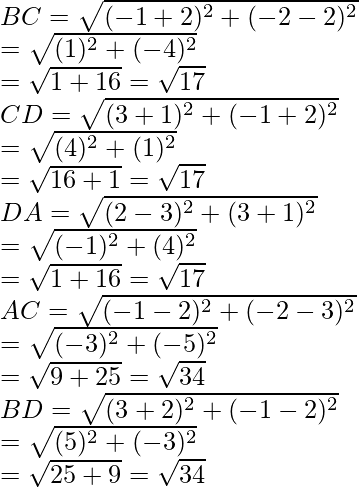
Therefore, AB, BC, CD and DA are equal and diagonals AC and BD are also equal

Hence, ABCD is a square.

(iii) Using distance formula

$PQ=\sqrt{(\sqrt{2}+\sqrt{2})^2+(\sqrt{2}+\sqrt{2})^2}\\ =\sqrt{(2\sqrt{2})^2+(2\sqrt{2})^2}=\sqrt{16}=4\\ PR=\sqrt{(\sqrt{2}+\sqrt{6})^2+(\sqrt{2}-\sqrt{6})^2} \\ =\sqrt{2+6+2\sqrt{12}+2+6-2\sqrt{12}}=\sqrt{16}=4\\ RQ=\sqrt{(-2\sqrt{2}+\sqrt{6})^2+(-2\sqrt{2}-\sqrt{6})^2}\\ =\sqrt{2+6-2\sqrt{12}+2+6+2\sqrt{12}}=\sqrt{16}=4$

Since, PQ = PR = RQ = 4, so, the point P, Q, R form an equilateral triangle.

Question 30. Find the point on x-axis which is equidistant from the points (-2, 5) and (2, -3).

Solution:

Let us considered point P lies on x-axis

So, the coordinates of point P be (x, 0)

It is given that P is equidistant from A (-2, 5) and B (2, -3)

$AP=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ =\sqrt{(x+2)^2+(0-5)^2}$

AP²= (x + 2)²+ (-5)²

= (x + 2)²+ 25

BP²= (x – 2)²+ (0 + 3)²

= (x – 2)²+ 9

AP = BP

So, AP²= BP²

(x + 2)²+ 25 = (x – 2)²+ 9

x²+ 4x + 4 + 25 = x²– 2x + 4 + 9

x²+ 4x – x²+ 4x = 13 – 29

8x = -16

x = -16/8

x = -2

Hence, the co-ordinates of point P are(-2, 0)

Question 31. Find the value of x such that PQ = QR where the co-ordinates of P, Q, and R are (6, -1), (1, 3), and (x, 8) respectively.

Solution:

Given that the co-ordinates of P (6, -1), Q(1, 3), and R(x, 8)

Also, PQ = QR

By using the distance formula

D = 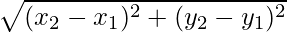$\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}$

We find the length of PQ and QR

PQ²= (1 – 6)²+ (3 + 1)²

= (-5)²+ (4)²

= 25 + 16 = 41

QR²= (x – 1)²+ (8 – 3)²

= (x – 1)²+ (5)²= (x – 1)²+ 25

It is given that PQ = QR

So PQ² = QR²

41 = (x – 1)²+ 25

(x – 1)²= 41 – 25 = 16 = (±4)²

x – 1 = ±4

If x – 1 = 4, then x = 1 + 4 = 5

If x – 1 = -4 then x = -4 + 1 = -3

Hence, the value of x = 5, -3

Question 32. Prove that the points (0, 0), (5, 5), and (-5, 5) are the vertices of a right isosceles triangle.

Solution:

Let us consider ABC is a triangle whose vertices are A(0, 0), B(5, 5), and C(-5, 5)

Now $AB=\sqrt{(x_2-x_1)^2(y_2-y_1)^2}\\ AB=\sqrt{(5-0)^2(5-0)^2}\\ =\sqrt{(5)^2(5)^2}=\sqrt{25+25}=\sqrt{50}$

AB²= (√50)²= 50

Similarly, BC²= (-5 – 5)²(5 – 5)²

= (-10)²+ (0)²= 100 + 0 = 100

and CA²= (0 + 5)²+ (0 – 5)²

= (5)²+ (-5)²= 25 + 25 = 50

Here, we conclude that AB = CA

Also, AB²+ CA²= 50 + 50 = 100 = BC²

Hence, the triangle ABC is a right triangle.

Question 33. If the points P (x, y) is equidistant from the points A (5, 1) and B (1,5), prove that x = y.

Solution:

Let us consider P(x, y) is equidistant from the points A (5, 1) and B (1,5),

It is given that PA = PB

Also, PA² = PB²

Now, PA=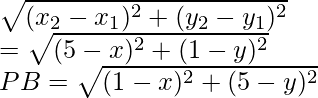 $\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ =\sqrt{(5-x)^2+(1-y)^2}\\ PB=\sqrt{(1-x)^2+(5-y)^2}\\$

On squaring both sides, we get

(5 – x)²+ (1 – y)²= (1 – x)²+ (5 – y)²

25 + x²– 10x + 1 + y²– 2y = 1 + x²– 2x + 25 + y²– 10y

-10x – 2y + 26 = -2x – 10y + 26

-10x – 2y = -2x – 10y

-10x + 2x = -10y + 2y

-8x = -8xy

x = y

Question 34. If Q (0, 1) is equidistant from P (5, -3) and R (x, 6) find the values of x. Also find the distances QR and PR.

Solution:

Given that Q (0, 1) is equidistant from P (5, -3) and R (x, 6)

So, PQ = RQ

Also, PA² = PB²

Now 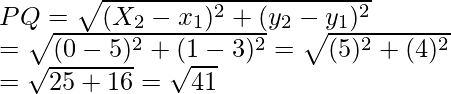$PQ=\sqrt{(X_2-x_1)^2+(y_2-y_1)^2}\\ =\sqrt{(0-5)^2+(1-3)^2}=\sqrt{(5)^2+(4)^2}\\ =\sqrt{25+16}=\sqrt{41}$

PQ²= 41

Similarly, RQ²= (-x)²+ (1 – 6)²

= (-x)²+ (-5)²= x²+ 25

It is given that PQ = RQ
So,

x²+ 25 = 41

x²= 41 – 25

x²= (±4)²

x = ±4

Now, QR = √x²+ 25 = √41

So, when x = 4, then

$PR=\sqrt{(5-4)^2+(-3-6)^2}\\ =\sqrt{(1)^2+(-9)^2}=\sqrt{1+18}=\sqrt{82}$

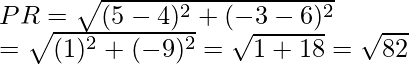
or when x = -4, then

$PR=\sqrt{(5+4)^2+(-3-6)^2}\\ =\sqrt{(9)^2+(-9)^2}=\sqrt{81+81}\\ \sqrt{81*2}=9\sqrt{2}$

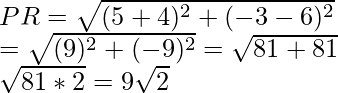
Question 35. Find the values of y for which the distance between the points P (2, -3) and Q (10, y) is 10 units.

Solution:

Given that the distance between P (2, -3) and Q (10, y) = 10

So, $PQ=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\\ =\sqrt{(10-2)^2+(y+3)^2}\\ =\sqrt{(8)^2+(y+3)^2}=\sqrt{64+(y+3)^2}\\ \sqrt{64+(y+3)^2}=10$

On squaring both side we get

64 + (y + 3)²= 100

(y + 3)²= 100 – 64

(y + 3)²= 36

y + 3 = (±6)²

So, when y + 3 = 6, then y = 6 – 3 = 3

Or when y + 3 = -6, then y = -6 – 3 = -9

Hence, y = 3, -9

Question 36. If the point P (k – 1, 2) is equidistant from the points A (3, k) and B (k, 5), find the values of k.

Solution:

Given that the point P (k – 1, 2) is equidistant from A (3, k) and B (k, 5)

So, PA = PB

Also, PA² = PB²

(k – 1 – 3)²+ (2 – k)²= (k – 1 – k)²+ (2 – 5)²

(k – 4)²+ (2 – k)²= (-1)²+ (-3)²

k²– 8k + 16 + 4 – 4k + k²= 1 + 9

2k²– 12k + 20 – 10 = 0

2k²– 12k + 10 = 0

k²– k – 5k + 5 = 0

k(k – 1) – 5(k – 5) = 0

(k – 1)(k – 5) = 0

Either k – 1 = 0, then k = 1

or k – 5 = 0, then k = 5

Hence, k = 1, 5

Question 37. If the point A (0, 2) is equidistant from the point B (3, p) and C (p, 5), find p. Also, find the length of AB.

Solution:

Given that point A (0, 2) is equidistant from B (3, p) and C (p, 5)

So, AB = AC

Also, AB² = AC²

(0 – 3)²+ (2 – p)²= (0 – p)²+ (2 – 5)²

9 + (2 – p)²= p²+ 9

9 + 4 + p²– 4p = p²+ 9

p²– 4p + 13 – p²– 9 = 0

-4p – 4 = 0

-4p = -4

p = -4/-4 = 1

p = 1

and AB = 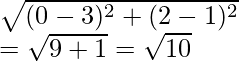$\sqrt{(0-3)^2+(2-1)^2}\\ =\sqrt{9+1}=\sqrt{10}$

Question 38. Name the quadrilateral formed, if any, by the following points, and give reasons for your answers :

(i) A (-1, -2), B (1, 0), C (-1, 2), D (-3, 0)

(ii) A (-3, 5), B (3, 1), C (0, 3), D (-1, -4)

(iii) A (4, 5), B (7, 6), C (4, 3), D (1, 2)

Solution:

(i) Given points are A (-1, -2), B (1, 0), C (-1, 2), D (-3, 0)

Now $AB=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}$

AB²= (x₂– x₁)² + (y₂– y₁)²

= (1 + 1)²+ (0 + 2)²

= (2)²+ (2)²

= 4 + 4 = 8

Similarly, BC²= (-1 – 1)²+ (2 – 0)²

= (-2)²+ (2)²

= 4 + 4 = 8

CD²= (-3 + 1)²+ (0 – 2)²

= (-2)²+ (-2)²

= 4 + 4 = 8

DA²= (-1 + 3)²+ (-2 + 0)²

= (2)²+ (-2)²

= 4 + 4 = 8

Diagonal AC²= (-1 + 1)²+ (2 + 2)²

= (0)²+ (4)²= 0 + 16 = 16

and BD²= (-3 – 1)²+ (0 – 0)²= (-4)²+ (0) = 16

Therefore, the sides are equal and diagonals are also equal

Hence, the quadrilateral ABCD is a square.

(ii) Given points are A (-3, 5), B (3, 1), C (0, 3), D (-1, -4)

Now AB $=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}$

AB²= (x₂– x₁)² + (y₂– y₁)²

= (3 + 3)²+ (1 – 5)²

= (6)²+ (-4)²

= 36 + 16 = 52

Similarly, BC²= (0 – 3)²+ (3 – 1)²

= (-3)²+ (2)²

= 9 + 4 = 13

CD²= (-1 – 0)²+ (-4 – 3)²

= (-1)²+ (-7)²

= 1 + 49 = 50

DA²= (-3 + 1)²+ (5 + 4)²

= (-2)²+ (9)²

= 4 + 81 = 85

Diagonal AC²= (0 + 3)²+ (3 – 5)²= (3)²+ (-2)² = 9 + 4 = 13

In triangle ABC

The length of AB = √52, AC = √13, BC = √13

AC + BC = √13 + √13 = 2√13

= √4 * 13 = √52

AC + BC = AB

Triangle ABC is not possible

Hence, ABCD is not a quadrilateral

(iii) Points A (4, 5), B (7, 6), C (4, 3), D (1, 2)

Now AB $=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}$

AB²= (x₂– x₁)² + (y₂– y₁)²

= (7 – 4)²+ (6 – 5)²

= (3)²+ (1)²

= 9 + 1 = 10

Similarly, BC²= (4 – 7)²+ (3 – 6)²

= (3)²+ (-3)²= 9 + 9 = 18

CD²= (1 – 4)²+ (2 – 3)²

= (-3)²+ (-1)²

= 9 + 1 = 10

DA²= (4 – 1)²+ (5 – 2)²= (3)²+ (3)² = 9 + 9 = 18

Here AB = CD and BC = DA

Diagonal AC²= (4 – 4)²+ (3 – 5)²

= (0)²+ (-2)²

= 0 + 4 = 4

and BD²= (1 – 7)²+ (2 – 6)²= (-6)²+ (-4)²

= 36 + 16 = 52

Hence the opposite sides are equal and diagonals are not equal.

So, ABCD is a parallelogram.

Question 39. Find the equation of the perpendicular bisector of the line segment joining points (7, 1) and (3, 5).

Solution:

Let the given points are A (7, 1) and B (3, 5) and mid point be M

Coordinates of mid-point AB = ((7 + 3)/2, (1 + 5)/2) = (5, 3)

Now slope of AB(m₁)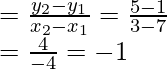 $=\frac{y_2-y_1}{x_2-x_1}=\frac{5-1}{3-7}\\ =\frac{4}{-4}=-1$

Slope of perpendicular to AB(m₂) = -1/m₁ = -(-1) = -1

So, the equation of the perpendicular line passing through multiple y – y₁= m(x – x₁)

y – 3 = 1(x – 5)

y – 3 = x – 5

x – y = -3 + 5

x – y = 2

Suggest improvement

Class 10 RD Sharma Solutions - Chapter 14 Coordinate Geometry - Exercise 14.2 | Set 1

Class 10 RD Sharma Solutions - Chapter 14 Coordinate Geometry - Exercise 14.2 | Set 3

Share your thoughts in the comments

Chapter 1: Real Numbers

Chapter 2: Polynomials

Chapter 3: Pair of Linear Equations in Two Variables

Chapter 4: Triangles

Chapter 5: Trigonometric Ratios

Chapter 6: Trigonometric Identities

Chapter 7: Statistics

Chapter 8: Quadratic Equations

Chapter 9: Arithmetic Progressions

Chapter 10: Circles

Chapter 11: Constructions

Chapter 12: Some Applications of Trigonometry

Chapter 13: Probability

Chapter 14: Coordinate Geometry

Chapter 15: Areas Related To Circles

Chapter 16: Surface Areas And Volumes

Chapter 1: Real Numbers

Chapter 2: Polynomials

Chapter 3: Pair of Linear Equations in Two Variables

Chapter 4: Triangles

Chapter 5: Trigonometric Ratios

Chapter 6: Trigonometric Identities

Chapter 7: Statistics

Chapter 8: Quadratic Equations

Chapter 9: Arithmetic Progressions

Chapter 10: Circles

Chapter 11: Constructions

Chapter 12: Some Applications of Trigonometry

Chapter 13: Probability

Chapter 14: Coordinate Geometry

Chapter 15: Areas Related To Circles

Chapter 16: Surface Areas And Volumes

Class 10 RD Sharma Solutions – Chapter 14 Coordinate Geometry – Exercise 14.2 | Set 2

Question 20. The three vertices of a parallelogram are (3, 4), (3, 8), and (9, 8). Find the fourth vertex.

Question 21. Find a point which is equidistant from the point A (-5, 4) and B (-1, 6). How many such points are there?

Question 22. The centre of a circle is (2a, a – 7). Find the values of a if the circle passes through the point (11, -9) and has diameter 10√2 units.

Question 24. Find the value of k, if the point P (0, 2) is equidistant from (3, k) and (k, 5).

Question 25. If (-4, 3) and (4, 3) are two vertices of an equilateral triangle, find the coordinates of the third vertex, given that the origin lies in the

Question 26. Show that the points (-3, 2), (-5, -5), (2, -3), and (4, 4) are the vertices of a rhombus. Find the area of this rhombus.

Question 27. Find the coordinates of the circumcenter of the triangle whose vertices are (3, 0), (-1, -6), and (4, -1). Also, find its circumradius.

Question 28. Find a point on the x-axis which is equidistant from the points (7, 6) and (-3, 4).

Question 29. (i) Show that the points A (5, 6), B (1, 5), C (2, 1), and D (6, 2) are the vertices of a square.

(ii) Prove that the points A (2, 3), B (-2, 2), C (-1, -2), and D (3, -1) are the vertices of a square ABCD.

(iii) Name the type of triangle PQR formed by the point P(√2, √2), Q(- √2, – √2), and R (-√6, √6).

Question 30. Find the point on x-axis which is equidistant from the points (-2, 5) and (2, -3).

Question 31. Find the value of x such that PQ = QR where the co-ordinates of P, Q, and R are (6, -1), (1, 3), and (x, 8) respectively.

Question 32. Prove that the points (0, 0), (5, 5), and (-5, 5) are the vertices of a right isosceles triangle.

Question 33. If the points P (x, y) is equidistant from the points A (5, 1) and B (1,5), prove that x = y.

Question 34. If Q (0, 1) is equidistant from P (5, -3) and R (x, 6) find the values of x. Also find the distances QR and PR.

Question 35. Find the values of y for which the distance between the points P (2, -3) and Q (10, y) is 10 units.

Question 36. If the point P (k – 1, 2) is equidistant from the points A (3, k) and B (k, 5), find the values of k.

Question 37. If the point A (0, 2) is equidistant from the point B (3, p) and C (p, 5), find p. Also, find the length of AB.

Question 38. Name the quadrilateral formed, if any, by the following points, and give reasons for your answers :

Question 39. Find the equation of the perpendicular bisector of the line segment joining points (7, 1) and (3, 5).

Please Login to comment...

Similar Reads

What kind of Experience do you want to share?