Class 12 NCERT Solutions- Mathematics Part I – Chapter 5 Continuity And Differentiability – Exercise 5.1

Question 1. Prove that the function f(x) = 5x – 3 is continuous at x = 0, at x = – 3 and at x = 5.

Solution:

To prove the continuity of the function f(x) = 5x – 3, first we have to calculate limits and function value at that point.

Continuity at x = 0

Left limit =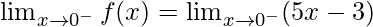 

= (5(0) – 3) = -3

Right limit =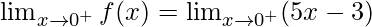 

= (5(0) – 3)= -3

Function value at x = 0, f(0) = 5(0) – 3 = -3

As, ,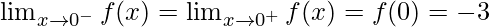 

Hence, the function is continuous at x = 0.

Continuity at x = -3

Left limit =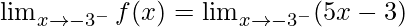 

= (5(-3) – 3) = -18

Right limit =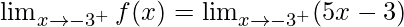 

= (5(-3) – 3) = -18

Function value at x = -3, f(-3) = 5(-3) – 3 = -18

As,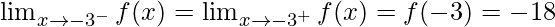 

Hence, the function is continuous at x = -3.

Continuity at x = 5

Left limit =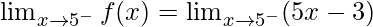 

= (5(5) – 3) = 22

Right limit =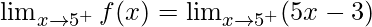 

= (5(5) – 3) = 22

Function value at x = 5, f(5) = 5(5) – 3 = 22

As,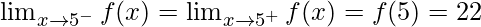 

Hence, the function is continuous at x = 5.

Question 2. Examine the continuity of the function f(x) = 2x² – 1 at x = 3.

Solution:

To prove the continuity of the function f(x) = 2x² – 1, first we have to calculate limits and function value at that point.

Continuity at x = 3

Left limit =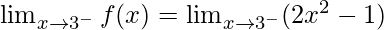 

= (2(3)² – 1) = 17

Right limit = 

= (2(3)² – 1) = 17

Function value at x = 3, f(3) = 2(3)² – 1 = 17

As, 

Hence, the function is continuous at x = 3.

Question 3. Examine the following functions for continuity.

(a) f(x) = x – 5

Solution:

To prove the continuity of the function f(x) = x – 5, first we have to calculate limits and function value at that point.

Let’s take a real number, c

Continuity at x = c

Left limit =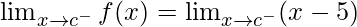 

= (c – 5) = c – 5

Right limit =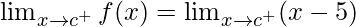 

= (c – 5) = c – 5

Function value at x = c, f(c) = c – 5

As, 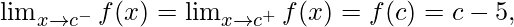for any real number c

Hence, the function is continuous at every real number.

(b) 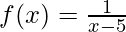, x ≠ 5

Solution:

To prove the continuity of the function f(x) = 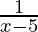, first we have to calculate limits and function value at that point.

Let’s take a real number, c

Continuity at x = c and c ≠ 5

Left limit =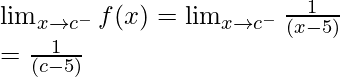 

Right limit =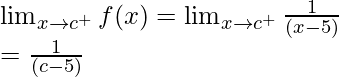 

Function value at x = c, f(c) =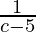 

As, 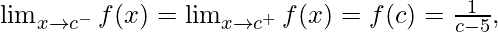for any real number c

Hence, the function is continuous at every real number.

(c) 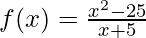, x ≠ -5

Solution:

To prove the continuity of the function f(x) = 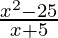, first we have to calculate limits and function value at that point.

Let’s take a real number, c

Continuity at x = c and c ≠ -5

Left limit =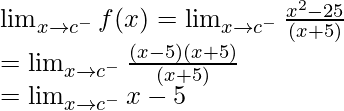 

= c – 5

Right limit =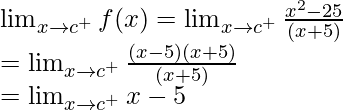 

= c – 5

Function value at x = c, f(c) =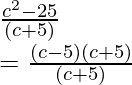 

= c – 5

As, 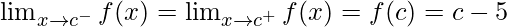, for any real number c

Hence, the function is continuous at every real number.

(d) f(x) = |x – 5|

Solution:

To prove the continuity of the function f(x) = |x – 5|, first we have to calculate limits and function value at that point.

Here,

As, we know that modulus function works differently.

In |x – 5|, |x – 5| = x – 5 when x>5 and |x – 5| = -(x – 5) when x < 5

Let’s take a real number, c and check for three cases of c:

Continuity at x = c

When c < 5

Left limit = 

= -(c – 5) 

= 5 – c

Right limit =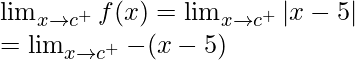 

= -(c – 5)

= 5 – c

Function value at x = c, f(c) = |c – 5| = 5 – c

As,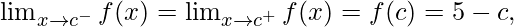 

Hence, the function is continuous at every real number c, where c<5.

When c > 5

Left limit =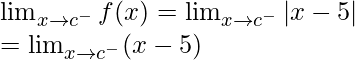 

= (c – 5)

Right limit =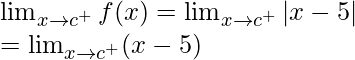 

= (c – 5)

Function value at x = c, f(c) = |c – 5| = c – 5

As, ,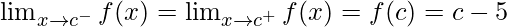

Hence, the function is continuous at every real number c, where c > 5.

When c = 5

Left limit =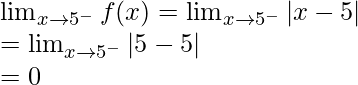 

Right limit =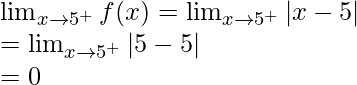 

Function value at x = c, f(c) = |5 – 5| = 0

As,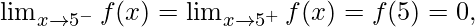 

Hence, the function is continuous at every real number c, where c = 5.

Hence, we can conclude that, the modulus function is continuous at every real number.

Question 4. Prove that the function f(x) = xⁿ is continuous at x = n, where n is a positive integer.

Solution:

To prove the continuity of the function f(x) = xⁿ, first we have to calculate limits and function value at that point.

Continuity at x = n

Left limit =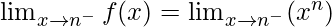 

= nⁿ

Right limit =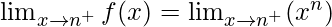 

= nⁿ

Function value at x = n, f(n) = nⁿ

As,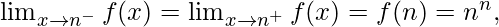 

Hence, the function is continuous at x = n.

Question 5. Is the function f defined by

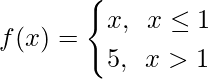
continuous at x = 0? At x = 1? At x = 2?

To prove the continuity of the function f(x), first we have to calculate limits and function value at that point.

Continuity at x = 0

Left limit =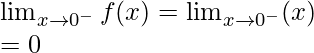 

Right limit =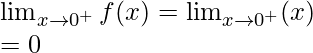 

Function value at x = 0, f(0) = 0

As,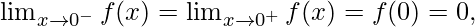 

Hence, the function is continuous at x = 0.

Continuity at x = 1

Left limit =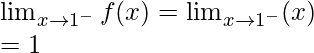 

Right limit =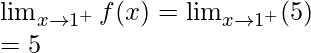 

Function value at x = 1, f(1) = 1

As, ,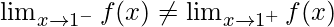

Hence, the function is not continuous at x = 1.

Continuity at x = 2

Left limit = 

Right limit =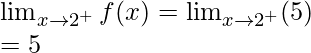 

Function value at x = 2, f(2) = 5

As, ,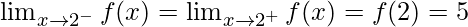

Therefore, the function is continuous at x = 2.

Find all points of discontinuity of f, where f is defined by

Question 6. 

Solution:

Here, as it is given that

For x ≤ 2, f(x) = 2x + 3, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (-∞, 2)

Now, For x > 2, f(x) = 2x – 3, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (2, ∞)

So now, as f(x) is continuous in x ∈ (-∞, 2) U (2, ∞) = R – {2}

Let’s check the continuity at x = 2,

Left limit =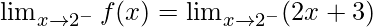 

= (2(2) + 3)

= 7

Right limit =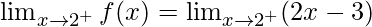 

= (2(2) – 3)

= 1

Function value at x = 2, f(2) = 2(3) + 3 = 7

As,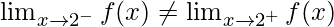 

Therefore, the function is discontinuous at only x = 2.

Question 7.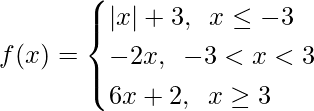 

Solution:

Here, as it is given that

For x ≤ -3, f(x) = |x| + 3, 

As, we know that modulus function works differently.

In |x|, |x – 0| = x when x > 0 and |x – 0| = -x when x < 0

f(x) = -x + 3, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (-∞, -3)

For -3 < x < 3, f(x) = -2x, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (-3, 3)

Now, for x ≥ 3, f(x) = 6x + 2, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (3, ∞)

So now, as f(x) is continuous in x ∈ (-∞, -3) U(-3, 3) U (3, ∞) = R – {-3, 3}

Let’s check the continuity at x = -3,

Left limit =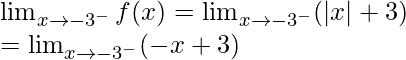 

= (-(-3) + 3)

= 6

Right limit =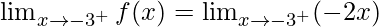 

= (-2(-3))

= 6

Function value at x = -3, f(-3) = |-3| + 3 = 3 + 3 = 6

As,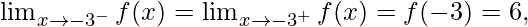 

Hence, the function is continuous at x = -3.

Now, let’s check the continuity at x = 3,

Left limit =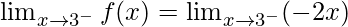 

= (-2(3))

= -6

Right limit = 

= (6(3) + 2)

= 20

Function value at x = 3, f(3) = 6(3) + 2 = 20

As,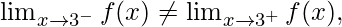 

Therefore, the function is discontinuous only at x = 3.

Question 8.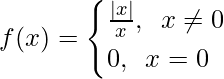 

Solution:

As, we know that modulus function works differently.

In |x|, |x – 0| = x when x > 0 and |x – 0|= -x when x < 0

When x < 0, f(x) = 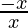= -1, which is a constant

As constant functions are continuous, hence f(x) is continuous x ∈ (-∞, 0).

When x > 0, f(x) = 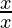= 1, which is a constant

As constant functions are continuous, hence f(x) is continuous x ∈ (0, ∞).

So now, as f(x) is continuous in x ∈ (-∞, 0) U(0, ∞) = R – {0}

Let’s check the continuity at x = 0,

Left limit =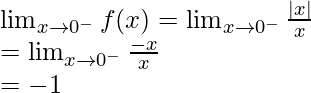 

Right limit =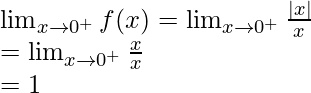 

Function value at x = 0, f(0) = 0

As,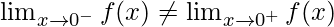 

Hence, the function is discontinuous at only x = 0.

Question 9.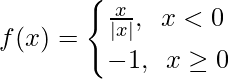 

Solution:

As, we know that modulus function works differently.

In |x|, |x – 0| = x when x > 0 and |x – 0| = -x when x < 0

When x < 0, f(x) = 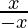= -1, which is a constant

As constant functions are continuous, hence f(x) is continuous x ∈ (-∞, 0).

When x > 0, f(x) = -1, which is a constant

As constant functions are continuous, hence f(x) is continuous x ∈ (0, ∞).

So now, as f(x) is continuous in x ∈ (-∞, 0) U(0, ∞) = R – {0}

Let’s check the continuity at x = 0,

Left limit =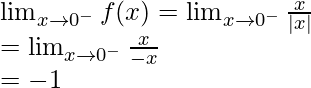 

Right limit =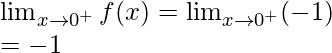 

Function value at x = 0, f(0) = -1

As,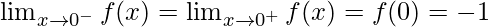 

Hence, the function is continuous at x = 0.

So, we conclude that the f(x) is continuous at any real number. Hence, no point of discontinuity.

Question 10.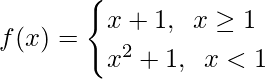 

Solution:

Here,

When x ≥1, f(x) = x + 1, which is a polynomial

As polynomial functions are continuous, hence f(x) is continuous x ∈ (1, ∞)

When x < 1, f(x) = x² + 1, which is a polynomial

As polynomial functions are continuous, hence f(x) is continuous x ∈ (-∞, 1)

So now, as f(x) is continuous in x ∈ (-∞, 1) U (1, ∞) = R – {1}

Let’s check the continuity at x = 1,

Left limit =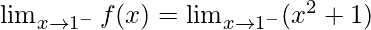 

= 1 + 1

= 2

Right limit =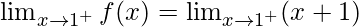 

= 1 + 1

= 2

Function value at x = 1, f(1) = 1 + 1 = 2

As,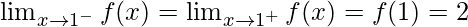 

Hence, the function is continuous at x = 1.

So, we conclude that the f(x) is continuous at any real number.

Question 11.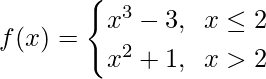 

Solution:

Here,

When x ≤ 2, f(x) = x³ + 3, which is a polynomial

As polynomial functions are continuous, hence f(x) is continuous x ∈ (-∞, 2)

When x > 2, f(x) = x² + 1, which is a polynomial

As polynomial functions are continuous, hence f(x) is continuous x ∈ (2, ∞)

So now, as f(x) is continuous in x ∈ (-∞, 2) U(2, ∞) = R – {2}

Let’s check the continuity at x = 2,

Left limit =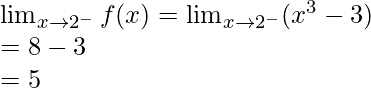 

Right limit =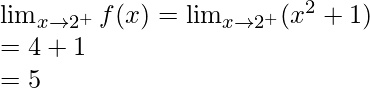 

Function value at x = 2, f(2) = 8 – 3 = 5

As, 

Hence, the function is continuous at x = 2.

So, we conclude that the f(x) is continuous at any real number.

Question 12.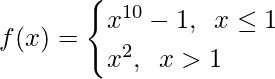 

Solution:

Here,

When x ≤ 1, f(x) = x¹⁰ – 1, which is a polynomial

As polynomial functions are continuous, hence f(x) is continuous x ∈ (-∞, 1)

When x >1, f(x) = x², which is a polynomial

As polynomial functions are continuous, hence f(x) is continuous x ∈ (1, ∞)

So now, as f(x) is continuous in x ∈ (-∞, 1) U (1, ∞) = R – {1}

Let’s check the continuity at x = 1,

Left limit = 

= 1 – 1

= 0

Right limit =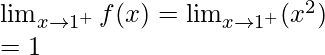 

Function value at x = 1, f(1) = 1 – 1 = 0

As,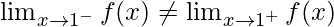 

Hence, the function is discontinuous at x = 1.

Question 13. Is the function defined by

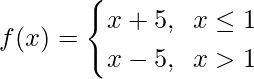
a continuous function?

Solution:

Here, as it is given that

For x ≤ 1, f(x) = x + 5, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (-∞, 1)

Now, For x > 1, f(x) = x – 5, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (1, ∞)

So now, as f(x) is continuous in x ∈ (-∞, 1) U (1, ∞) = R – {1}

Let’s check the continuity at x = 1,

Left limit =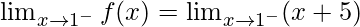 

= (1 + 5)

= 6

Right limit =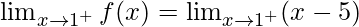 

= (1 – 5)

= -4

Function value at x = 1, f(1) = 5 + 1 = 6

As,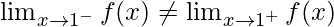 

Hence, the function is continuous for only R – {1}.

Discuss the continuity of the function f, where f is defined by

Question 14.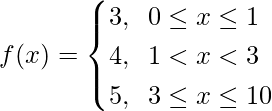 

Solution:

Here, as it is given that

For 0 ≤ x ≤ 1, f(x) = 3, which is a constant

As constants are continuous, hence f(x) is continuous x ∈ (0, 1)

Now, For 1 < x < 3, f(x) = 4, which is a constant 

As constants are continuous, hence f(x) is continuous x ∈ (1, 3)

For 3 ≤ x ≤ 10, f(x) = 5, which is a constant

As constants are continuous, hence f(x) is continuous x ∈ (3, 10)

So now, as f(x) is continuous in x ∈ (0, 1) U (1, 3) U (3, 10) = (0, 10) – {1, 3}

Let’s check the continuity at x = 1,

Left limit =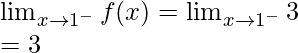 

Right limit = 

Function value at x = 1, f(1) = 3

As,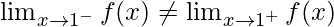 

Hence, the function is discontinuous at x = 1.

Now, let’s check the continuity at x = 3,

Left limit =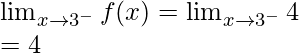 

Right limit =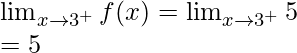 

Function value at x = 3, f(3) = 4

As,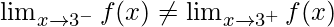 

Hence, the function is discontinuous at x = 3.

So concluding the results, we get

Therefore, the function f(x) is discontinuous at x = 1 and x = 3.

Question 15.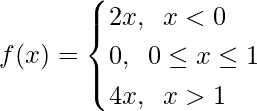 

Solution:

Here, as it is given that

For x < 0, f(x) = 2x, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (-∞, 0)

Now, For 0 ≤ x ≤ 1, f(x) = 0, which is a constant

As constant are continuous, hence f(x) is continuous x ∈ (0, 1)

For x > 1, f(x) = 4x, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (1, ∞)

So now, as f(x) is continuous in x ∈ (-∞, 0) U (0, 1) U (1, ∞)= R – {0, 1}

Let’s check the continuity at x = 0,

Left limit = 

Right limit =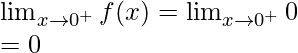 

Function value at x = 0, f(0) = 0

As,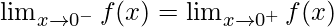 

Hence, the function is continuous at x = 0.

Now, let’s check the continuity at x = 1,

Left limit =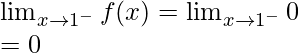 

Right limit =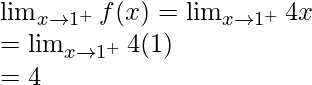 

Function value at x = 1, f(1) = 0

As,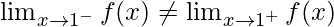 

Hence, the function is discontinuous at x = 1.

Therefore, the function is continuous for only R – {1}

Question 16.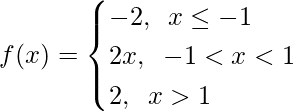 

Solution:

Here, as it is given that

For x ≤ -1, f(x) = -2, which is a constant

As constant are continuous, hence f(x) is continuous x ∈ (-∞, -1)

Now, For -1 ≤ x ≤ 1, f(x) = 2x, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (-1, 1)

For x > 1, f(x) = 2, which is a constant

As constant are continuous, hence f(x) is continuous x ∈ (1, ∞)

So now, as f(x) is continuous in x ∈ (-∞, -1) U (-1, 1) U (1, ∞)= R – {-1, 1}

Let’s check the continuity at x = -1,

Left limit =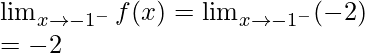 

Right limit =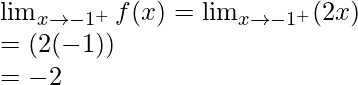 

Function value at x = -1, f(-1) = -2

As, 

Hence, the function is continuous at x = -1.

Now, let’s check the continuity at x = 1,

Left limit =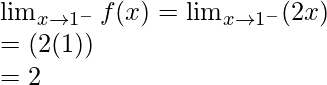 

Right limit =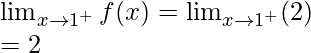 

Function value at x = 1, f(1) = 2(1) = 2

As,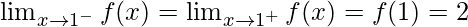 

Hence, the function is continuous at x = 1.

Therefore, the function is continuous for any real number.

Question 17. Find the relationship between a and b so that the function f defined by

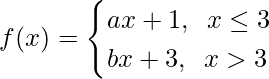
is continuous at x = 3.

Solution:

As, it is given that the function is continuous at x = 3.

It should satisfy the following at x = 3:

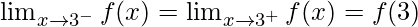
Continuity at x = 3,

Left limit =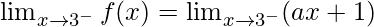 

= (a(3) + 1)

= 3a + 1

Right limit =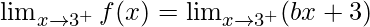 

= (b(3) + 3)

= 3b + 3

Function value at x = 3, f(3) = a(3) + 1 = 3a + 1

So equating both the limits, we get

3a + 1 = 3b + 3

3(a – b) = 2

a – b = 2/3

Question 18. For what value of λ is the function defined by

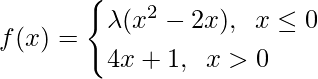
continuous at x = 0? What about continuity at x = 1?

Solution:

To be continuous function, f(x) should satisfy the following at x = 0:

Continuity at x = 0,

Left limit =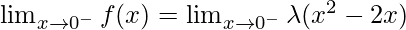 

= λ(0²– 2(0)) = 0

Right limit = 

= λ4(0) + 1 = 1

Function value at x = 0, f(0) =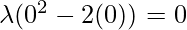 

As, 0 = 1 cannot be possible

Hence, for no value of λ, f(x) is continuous.

But here,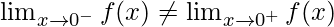 

Continuity at x = 1,

Left limit =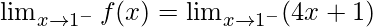 

= (4(1) + 1) = 5

Right limit =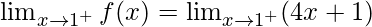 

= 4(1) + 1 = 5

Function value at x = 1, f(1) = 4(1) + 1 = 5

As,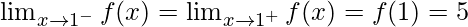 

Hence, the function is continuous at x = 1 for any value of λ.

Question 19. Show that the function defined by g (x) = x – [x] is discontinuous at all integral points. Here [x] denotes the greatest integer less than or equal to x. 

Solution:

[x] is greatest integer function which is defined in all integral points, e.g.

[2.5] = 2

[-1.96] = -2

x-[x] gives the fractional part of x.

e.g: 2.5 – 2 = 0.5

c be an integer

Let’s check the continuity at x = c,

Left limit = 

= (c – (c – 1)) = 1

Right limit = 

= (c – c) = 0

Function value at x = c, f(c) = c – = c – c = 0

As,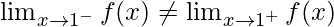 

Hence, the function is discontinuous at integral.

c be not an integer

Let’s check the continuity at x = c,

Left limit = 

= (c – (c – 1)) = 1

Right limit = 

= (c – (c – 1)) = 1

Function value at x = c, f(c) = c – = c – (c – 1) = 1

As,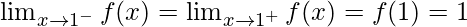 

Hence, the function is continuous at non-integrals part.

Question 20. Is the function defined by f(x) = x² – sin x + 5 continuous at x = π?

Solution:

Let’s check the continuity at x = π,

f(x) = x² – sin x + 5

Let’s substitute, x = π+h

When x⇢π, Continuity at x = π

Left limit =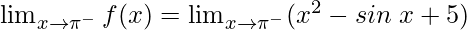 

= (π² – sinπ + 5) = π² + 5

Right limit =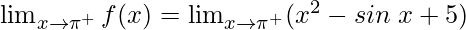 

= (π² – sinπ + 5) = π² + 5

Function value at x = π, f(π) = π² – sin π + 5 = π² + 5

As,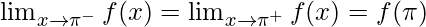 

Hence, the function is continuous at x = π .

Question 21. Discuss the continuity of the following functions:

(a) f(x) = sin x + cos x 

Solution:

Here, 

f(x) = sin x + cos x

Let’s take, x = c + h

When x⇢c then h⇢0

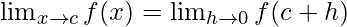
So, 

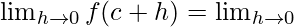(sin(c + h) + cos(c + h))

Using the trigonometric identities, we get

sin(A + B) = sin A cos B + cos A sin B

cos(A + B) =  cos A cos B – sin A sin B

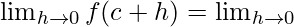((sinc cosh + cosc sinh) + (cosc cosh − sinc sinh))

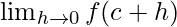= ((sinc cos0 + cosc sin0) + (cosc cos0 − sinc sin0))

cos 0 = 1 and sin 0 = 0

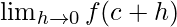 = (sinc + cosc) = f(c)

Function value at x = c, f(c) = sinc + cosc

As, 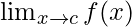= f(c) = sinc + cosc

Hence, the function is continuous at x = c.

(b) f(x) = sin x – cos x

Solution:

Here,

f(x) = sin x – cos x

Let’s take, x = c+h

When x⇢c then h⇢0

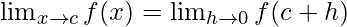
So,

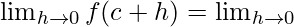(sin(c + h) − cos(c + h))

Using the trigonometric identities, we get

sin(A + B) = sin A cos B + cos A sin B

cos(A + B) =  cos A cos B – sin A sin B

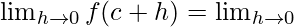((sinc cosh + cosc sinh) − (cosc cosh − sinc sinh))

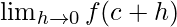 = ((sinc cos0 + cosc sin0) − (cosc cos0 − sinc sin0))

cos 0 = 1 and sin 0 = 0

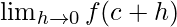 = (sinc − cosc) = f(c)

Function value at x = c, f(c) = sinc − cosc

As,  = f(c) = sinc − cosc

Hence, the function is continuous at x = c.

(c) f(x) = sin x . cos x

Solution:

Here,

f(x) = sin x + cos x

Let’s take, x = c+h

When x⇢c then h⇢0

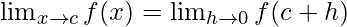
So,

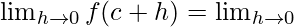sin(c + h) × cos(c + h))

Using the trigonometric identities, we get

sin(A + B) = sin A cos B + cos A sin B

cos(A + B) =  cos A cos B – sin A sin B

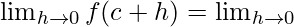((sinc cosh + cosc sinh) × (cosc cosh − sinc sinh))

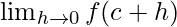= ((sinc cos0 + cosc sin0) × (cosc cos0 − sinc sin0))

cos 0 = 1 and sin 0 = 0

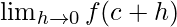 = (sinc × cosc) = f(c)

Function value at x = c, f(c) = sinc × cosc

As, 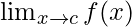= f(c) = sinc × cosc

Hence, the function is continuous at x = c.

Question 22. Discuss the continuity of the cosine, cosecant, secant and cotangent functions.

Solution:

Continuity of cosine

Here,

f(x) = cos x

Let’s take, x = c+h

When x⇢c then h⇢0

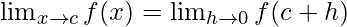
So,

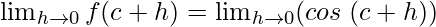
Using the trigonometric identities, we get

cos(A + B) =  cos A cos B – sin A sin B

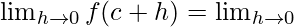(cosc cosh − sinc sinh)

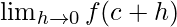 = (cosc cos0 − sinc sin0)

cos 0 = 1 and sin 0 = 0

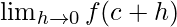 = (cosc) = f(c)

Function value at x = c, f(c) = (cosc)

As,  = f(c) = (cosc)

Hence, the cosine function is continuous at x = c.

Continuity of cosecant

Here,

f(x) = cosec x = 

Domain of cosec is R – {nπ}, n ∈ Integer

Let’s take, x = c + h

When x⇢c then h⇢0

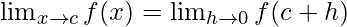
So,

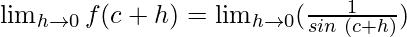
Using the trigonometric identities, we get

sin(A + B) = sin A cos B + cos A sin B

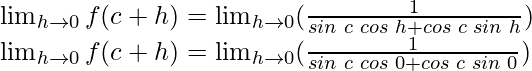
cos 0 = 1 and sin 0 = 0

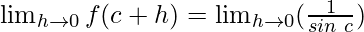
Function value at x = c, f(c) =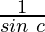 

As,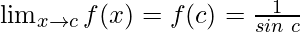 

Hence, the cosecant function is continuous at x = c.

Continuity of secant

Here,

f(x) = sec x =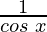 

Let’s take, x = c + h

When x⇢c then h⇢0

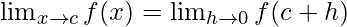
So,

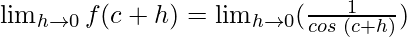
Using the trigonometric identities, we get

cos(A + B) =  cos A cos B – sin A sin B

cos 0 = 1 and sin 0 = 0

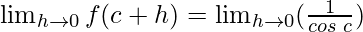
Function value at x = c, f(c) =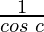 

As,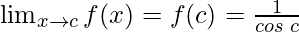 

Hence, the secant function is continuous at x = c.

Continuity of cotangent

Here,

f(x) = cot x =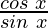 

Let’s take, x = c+h

When x⇢c then h⇢0

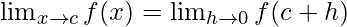
So,

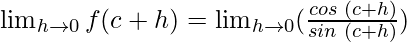
Using the trigonometric identities, we get

sin(A + B) = sin A cos B + cos A sin B

cos(A + B) =  cos A cos B – sin A sin B

cos 0 = 1 and sin 0 = 0

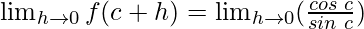
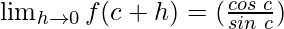
Function value at x = c, f(c) =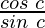 

As, 

Hence, the cotangent function is continuous at x = c.

Question 23. Find all points of discontinuity of f, where

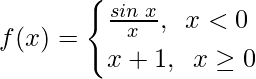
Solution:

Here,

From the two continuous functions g and h, we get

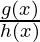= continuous when h(x) ≠ 0

For x < 0, f(x) = 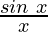, is continuous

Hence, f(x) is continuous x ∈ (-∞, 0)

Now, For x ≥ 0, f(x) = x + 1, which is a polynomial

As polynomial are continuous, hence f(x) is continuous x ∈ (0, ∞)

So now, as f(x) is continuous in x ∈ (-∞, 0) U (0, ∞)= R – {0}

Let’s check the continuity at x = 0,

Left limit =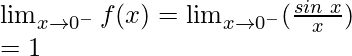 

Right limit =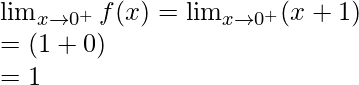 

Function value at x = 0, f(0) = 0 + 1 = 1

As,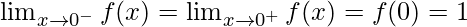 

Hence, the function is continuous at x = 0.

Hence, the function is continuous for any real number.

Question 24. Determine if f defined by

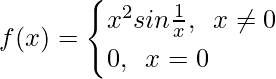
is a continuous function?

Solution:

Here, as it is given that

For x = 0, f(x) = 0, which is a constant

As constant are continuous, hence f(x) is continuous x ∈ = R – {0}

Let’s check the continuity at x = 0,

As, we know range of sin function is [-1,1]. So, -1 ≤ 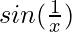≤ 1 which is a finite number.

Limit =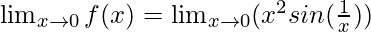 

= (0² ×(finite number)) = 0

Function value at x = 0, f(0) = 0

As,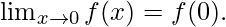 

Hence, the function is continuous for any real number.

Question 25. Examine the continuity of f, where f is defined by

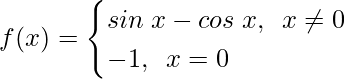
Solution:

Continuity at x = 0,

Left limit =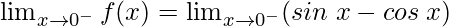 

= (sin0 − cos0) = 0 − 1 = −1

Right limit =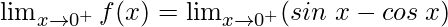 

= (sin0 − cos0) = 0 − 1 = −1

Function value at x = 0, f(0) = sin 0 – cos 0 = 0 – 1 = -1

As,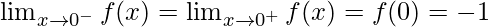 

Hence, the function is continuous at x = 0.

Continuity at x = c (real number c≠0),

Left limit =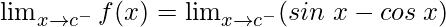 

= (sinc − cosc)

Right limit =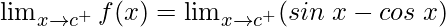 

= (sinc − cosc)

Function value at x = c, f(c) = sin c – cos c

As,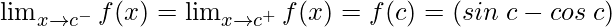 

So concluding the results, we get

The function f(x) is continuous at any real number.

Find the values of k so that the function f is continuous at the indicated point in Exercises 26 to 29. 

Question 26. 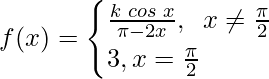 at x = π/2.

Solution:

Continuity at x = π/2

Let’s take x =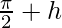 

When x⇢π/2 then h⇢0

Substituting x = 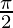+h, we get

cos(A + B) = cos A cos B – sin A sin B

Limit =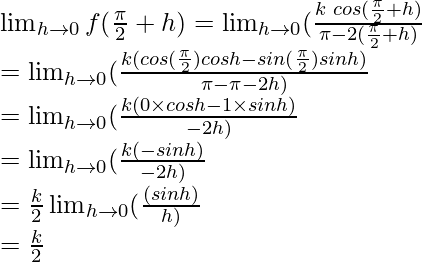 

Function value at x = 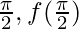= 3

As, 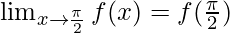should satisfy, for f(x) being continuous

k/2 = 3

k = 6

Question 27. 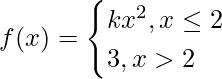 at x = 2

Solution:

Continuity at x = 2

Left limit =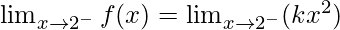 

= k(2)² = 4k

Right limit =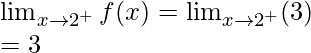 

Function value at x = 2, f(2) = k(2)² = 4k

As, 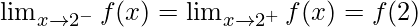 should satisfy, for f(x) being continuous

4k = 3

k = 3/4

Question 28. 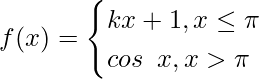 at x = π

Solution:

Continuity at x = π

Left limit =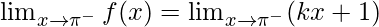 

= k(π) + 1

Right limit =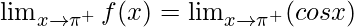 

= cos(π) = -1

Function value at x = π, f(π) = k(π) + 1

As,  should satisfy, for f(x) being continuous

kπ + 1 = -1

k = -2/π

Question 29. 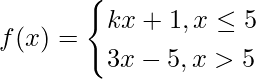 at x = 5

Solution:

Continuity at x = 5

Left limit =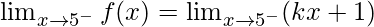 

= k(5) + 1 = 5k + 1

Right limit =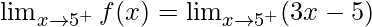 

= 3(5) – 5 = 10

Function value at x = 5, f(5) = k(5) + 1 = 5k + 1

As, 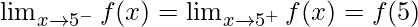should satisfy, for f(x) being continuous

5k + 1 = 10

k = 9/5 

Question 30. Find the values of a and b such that the function defined by

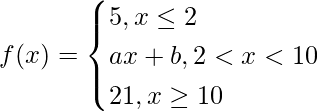
is a continuous function

Solution:

Continuity at x = 2

Left limit = 

Right limit =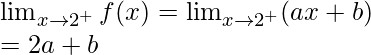 

Function value at x = 2, f(2) = 5

As, 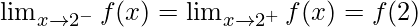should satisfy, for f(x) being continuous at x = 2

2a + b = 5 ……………………(1)

Continuity at x = 10

Left limit =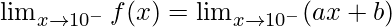 

= 10a + b

Right limit =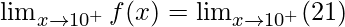 

= 21

Function value at x = 10, f(10) = 21

As, 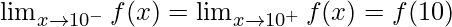should satisfy, for f(x) being continuous at x = 10

10a + b = 21 ……………………(2)

Solving the eq(1) and eq(2), we get

a = 2

b = 1

Question 31. Show that the function defined by f(x) = cos (x²) is a continuous function

Solution:

Let’s take

g(x) = cos x

h(x) = x²

g(h(x)) = cos (x²)

To prove g(h(x)) continuous, g(x) and h(x) should be continuous.

Continuity of g(x) = cos x

Let’s check the continuity at x = c

x = c + h

g(c + h) = cos (c + h)

When x⇢c then h⇢0

cos(A + B) =  cos A cos B – sin A sin B

Limit = 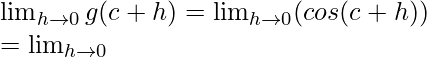(cosc cosh − sinc sinh)

= cosc cos0 − sinc sin0 = cosc

Function value at x = c, g(c) = cos c

As, 

The function g(x) is continuous at any real number.

Continuity of h(x) = x²

Let’s check the continuity at x = c

Limit =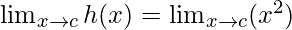 

= c²

Function value at x = c, h(c) = c²

As,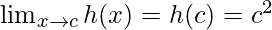 

The function h(x) is continuous at any real number.

As, g(x) and h(x) is continuous then g(h(x)) = cos(x²) is also continuous.

Question 32. Show that the function defined by f(x) = | cos x | is a continuous function. 

Solution:

Let’s take

g(x) = |x|

m(x) = cos x

g(m(x)) = |cos x|

To prove g(m(x)) continuous, g(x) and m(x) should be continuous.

Continuity of g(x) = |x|

As, we know that modulus function works differently.

In |x – 0|, |x| = x when x ≥ 0 and |x| = -x when x < 0

Let’s check the continuity at x = c

When c < 0

Limit =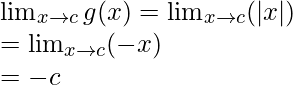 

Function value at x = c, g(c) = |c| = -c

As,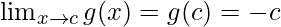 

When c ≥ 0

Limit =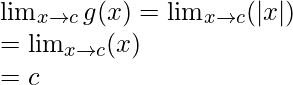 

Function value at x = c, g(c) = |c| = c

As, 

The function g(x) is continuous at any real number.

Continuity of m(x) = cos x

Let’s check the continuity at x = c

x = c + h

m(c + h) = cos (c + h)

When x⇢c then h⇢0

cos(A + B) =  cos A cos B – sin A sin B

Limit = 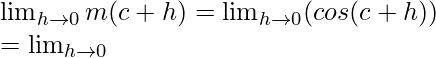(cosc cosh − sinc sinh)

= cosc cos0 − sinc sin0 = cosc

Function value at x = c, m(c) = cos c

As,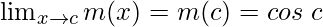 

The function m(x) is continuous at any real number.

As, g(x) and m(x) is continuous then g(m(x)) = |cos x| is also continuous.

Question 33. Examine that sin | x | is a continuous function.

Solution:

Let’s take

g(x) = |x|

m(x) = sin x

m(g(x)) = sin |x|

To prove m(g(x)) continuous, g(x) and m(x) should be continuous.

Continuity of g(x) = |x|

As, we know that modulus function works differently.

In |x-0|, |x|=x when x≥0 and |x|=-x when x<0

Let’s check the continuity at x = c

When c < 0

Limit =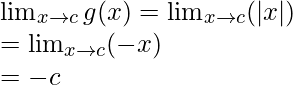 

Function value at x = c, g(c) = |c| = -c

As,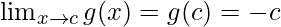 

When c ≥ 0

Limit =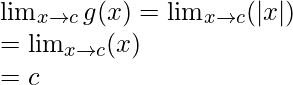 

Function value at x = c, g(c) = |c| = c

As, 

The function g(x) is continuous at any real number.

Continuity of m(x) = sin x

Let’s check the continuity at x = c

x = c + h

m(c + h) = sin (c + h)

When x⇢c then h⇢0

sin(A + B) = sin A cos B + cos A sin B

Limit = 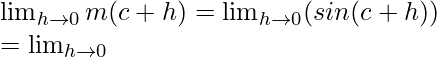(sinc cosh + cosc sinh) 

= sinc cos0 + cos csin0 = sinc

Function value at x = c, m(c) = sin c

As,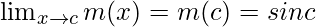 

The function m(x) is continuous at any real number.

As, g(x) and m(x) is continuous then m(g(x)) = sin |x| is also continuous.

Question 34. Find all the points of discontinuity of f defined by f(x) = | x | – | x + 1 |

Solution:

Let’s take

g(x) = |x|

m(x) = |x + 1|

g(x) – m(x) = | x | – | x + 1 |

To prove g(x) – m(x) continuous, g(x) and m(x) should be continuous.

Continuity of g(x) = |x|

As, we know that modulus function works differently.

In |x – 0|, |x| = x when x≥0 and |x| = -x when x < 0

Let’s check the continuity at x = c

When c < 0

Limit =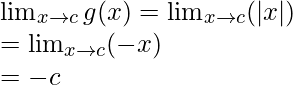 

Function value at x = c, g(c) = |c| = -c

As,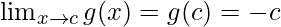 

When c ≥ 0

Limit =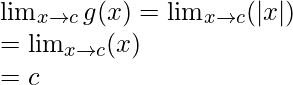 

Function value at x = c, g(c) = |c| = c

As, 

The function g(x) is continuous at any real number.

Continuity of m(x) = |x + 1|

As, we know that modulus function works differently.

In |x + 1|, |x + 1| = x + 1 when x ≥ -1 and |x + 1| = -(x + 1) when x < -1

Let’s check the continuity at x = c

When c < -1

Limit =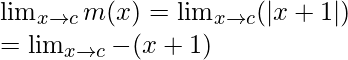 

= -(c + 1)

Function value at x = c, m(c) = |c + 1| = -(c + 1)

As,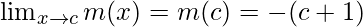 

When c ≥ -1

Limit = 

= c + 1

Function value at x = c, m(c) = |c| = c + 1

As,  = m(c) = c + 1

The function m(x) is continuous at any real number.

As, g(x) and m(x) is continuous then g(x) – m(x) = |x| – |x + 1| is also continuous.