Open In App

Motion of Center of Mass

Last Updated : 19 Apr, 2024
Improve
Improve
Like Article
Like
Save
Share
Report

Center of Mass is an important property of any rigid body system. Usually, these systems contain more than one particle. It becomes essential to analyze these systems as a whole. To perform calculations of mechanics, these bodies must be considered as a single-point mass. The Center of mass denotes such a point. Often the mechanical systems move in a transitory or a rotatory manner. In that case, the Center of mass also moves and acquires some velocity and acceleration. Let’s see how to calculate these metrics for such systems in detail. 

Center of Mass

A lot of problems can be simplified if it is assumed that the mass of the object is located at one particular point. If the correct position is chosen, then equations of forces and motion behave the same way as they behave if applied when mass is spread out. This special location is termed the Center of Mass.

Its position is defined relative to an object or the system of objects whose Center of mass is to be calculated. Usually for uniform shapes, it’s their centroid. For the shapes that are symmetrical and uniform, their Center of mass is located at their centroid. For a ring, its Center of mass lies inside the ring, which means the Center of mass of a body doesn’t need to lie in the body itself. 

Finding the Center of Mass

Now, it is clear that bodies that are uniform and symmetrical have their Center of masses at their centroid. But for bodies that are not symmetrical and uniform, the answer is not that simple. The Center of mass for such bodies can be anywhere. To work out the Center of mass of a complex object. A weighted average of the locations of each mass of the body is taken. 

Let’s say there is a body consisting of a set of masses “mi“, each at position ri, the location of the Center of mass rcm is given by the formula below. 

Mrcm = m1r1 + m2r2 + …. 

⇒ rcm = 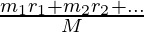\frac{ m_1r_1 + m_2r_2 + ...}{M}

In this case, M =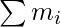 \sum m_i , which is the total mass of the body. 

The above technique uses vector arithmetic. To avoid vector arithmetic, we can find out the Center of mass of the body along the x-axis and y-axis respectively. Formulas for this case are given below: 

xcm = 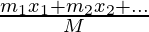\frac{ m_1x_1 + m_2x_2 + ...}{M}

ycm = 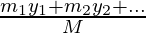\frac{ m_1y_1 + m_2y_2 + ...}{M}

Center of Gravity

Usually, gravity is assumed to be a uniform force acting on the body. The Center of gravity is the point at which gravity is assumed to be acting on the body. The Center of gravity is thus at the same place as the Center of mass. In physics literature, the terms Center of gravity and Center of mass are used interchangeably. They mean the same thing. 

Motion of Center of Mass

Consider a system of multiple particles. Each particle of that system is moving at a different velocity. How would someone assign a velocity to the system as a whole? Let us consider a system of particles m1, m2, m3 …and so on. The initial position vectors of these particles are r1, r2, r3 …rn. Now, these particles start moving in the directions of their position vectors. The goal is to find the velocity and the direction of the velocity of the Center of mass of the system. 

From the definition of the Center of mass, 

Mrcm = m1r1 + m2r2 + …. 

Since the particles are in motion, they are changing their position vectors. Differentiating the equation from both sides. 

\frac{d}{dr}(M\vec{r}) = \frac{d}{dr}(m_1\vec{r}_1 + m_1\vec{r}_2 + m_1\vec{r}_3 + ....m_1\vec{r}_n)

⇒ M\frac{d}{dr}(\vec{r}) = m_1\frac{d}{dr}(\vec{r}_1) + m_2\frac{d}{dr}(\vec{r}_2) + m_3\frac{d}{dr}\vec{r}_3 + ....

⇒ M\vec{v} = m_1\vec{v_1} + m_2\vec{v_2} + m_3\vec{v_3}+ ....

⇒\vec{v} = \frac{m_1\vec{v_1} + m_2\vec{v_2} + m_3\vec{v_3}+ ....}{M}

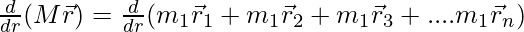
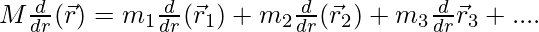
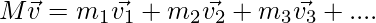
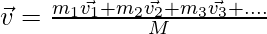
Similarly, if the particles are under acceleration. The equation given above can be differentiated again to find the acceleration of the Center of mass of the body. 

\vec{a} = \frac{m_1\vec{a_1} + m_2\vec{a_2} + m_3\vec{a_3}+ ....}{M}

Sample Problems

Question 1: Two point masses, m1 = 2Kg and m2 = 2Kg, are located moving at a speed of v1 = 2 m/s and v2 = 4 m/s respectively. Find the velocity of the Center of mass. 

Solution: 

The formula for the velocity of the Center of mass is given by, 

\vec{v} = \frac{m_1\vec{v_1} + m_2\vec{v_2} + m_3\vec{v_3}+ ....}{M}

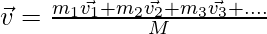
m1 = 2Kg, m2 = 2Kg and v1 = 2 m/s and v2 = 6 m/s. 

M = m1 + m2

⇒ M = 2 + 2 = 4

\vec{v} = \frac{m_1\vec{v_1} + m_2\vec{v_2} + m_3\vec{v_3}+ ....}{M}

⇒ \vec{v} = \frac{m_1\vec{v_1} + m_2\vec{v_2} }{M}

⇒ \vec{v} = \frac{(2)(2) + (2)(4) }{4}

⇒\vec{v} = 3 \text{ m/s}

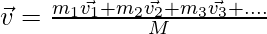
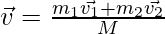
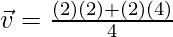
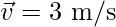
Question 2: Two-point masses, m1 = 10Kg and m2 = 20Kg, are located moving at a speed of v1 = 10 m/s and v2 = 5 m/s respectively. Find the velocity of the Center of mass. 

Solution: 

The formula for the velocity of the Center of mass is given by, 

\vec{v} = \frac{m_1\vec{v_1} + m_2\vec{v_2} + m_3\vec{v_3}+ ....}{M}

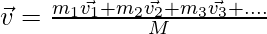
m1 = 10Kg,  m2 = 20Kg and v1 = 10 m/s and v2 = 5 m/s. 

M = m1 + m2

⇒ M = 10 + 20 = 30

\vec{v} = \frac{m_1\vec{v_1} + m_2\vec{v_2} + m_3\vec{v_3}+ ....}{M}

⇒ \vec{v} = \frac{m_1\vec{v_1} + m_2\vec{v_2} }{M}

⇒ \vec{v} = \frac{(10)(10) + (20)(5) }{30}

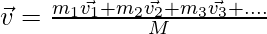
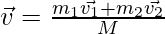
⇒\vec{v} = 20/3 m/s

Question 3: Two–point masses, m1 = 1Kg and m2 = 2Kg, have velocity vectors a = 6i + 4j and vector b = -5i + 2j respectively. Find the velocity of the Center of mass. 

Solution: 

The formula for the velocity of the Center of mass is given by, 

\vec{v} = \frac{m_1\vec{v_1} + m_2\vec{v_2} + m_3\vec{v_3}+ ....}{M}

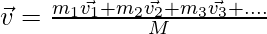
m1 = 1Kg, m2 = 2Kg and a = 6i + 4j, b = -5i + 2j

M = m1 + m2

⇒ M = 1 + 2 = 3

vcm = 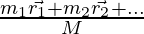\frac{ m_1\vec{r_1} + m_2\vec{r_2} + ...}{M}

⇒vcm = 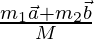\frac{ m_1\vec{a} + m_2\vec{b}}{M}

⇒ vcm = 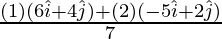\frac{ (1)(6\hat{i} + 4\hat{j} ) + (2)(-5\hat{i} + 2\hat{j})}{7}

⇒ vcm = 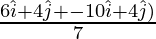\frac{ 6\hat{i} + 4\hat{j} + -10\hat{i} + 4\hat{j})}{7}

⇒ vcm = 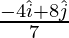\frac{ -4\hat{i} + 8\hat{j} }{7}

Question 4: Two–point masses, m1 = 4Kg and m2 = 2Kg, are located moving with velocity vectors a = i + j and vector b = -i + j respectively. Find the Center of mass. 

Solution: 

The formula for the velocity of Center of mass in the vector notation is given by, 

vcm = 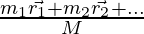\frac{ m_1\vec{r_1} + m_2\vec{r_2} + ...}{M}

m1 = 4Kg, m2 = 2Kg and a = i + j, b = -i + j

M = m1 + m2

⇒ M = 4 + 2 = 6

vcm = 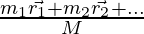\frac{ m_1\vec{r_1} + m_2\vec{r_2} + ...}{M}

⇒vcm = 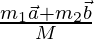\frac{ m_1\vec{a} + m_2\vec{b}}{M}

⇒ vcm = 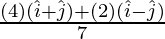\frac{ (4)(\hat{i} + \hat{j} ) + (2)(\hat{i} -\hat{j})}{7}

⇒ vcm = 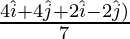\frac{ 4\hat{i} + 4\hat{j} + 2\hat{i} -2\hat{j})}{7}

⇒ vcm = 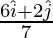\frac{ 6\hat{i} + 2\hat{j} }{7}

Question 5: A system consisting of two masses has a momentum of 16Kgm/s and its Center of mass has a velocity of 2 m/s. 4 Kg mass has a velocity of 4 m/s. Find the velocity of the other mass. 

Solution: 

The formula for the velocity of the Center of mass in the vector notation is given by, 

vcm = 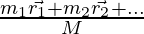\frac{ m_1\vec{r_1} + m_2\vec{r_2} + ...}{M}

m2 = ?, m1 = 4Kg, v2 = ? And v1 = 4 m/s.

M = m1 + m2

⇒ M = 4 + m = 4 + m

Mvcm = 16

⇒ (m + 4)(2) = 16 

⇒ m + 4 = 8 

⇒m = 4Kg

Thus, m2 = 4Kg. 

Mvcm = m1v1 + m2v2

⇒2(8) = (4)(4) + (4)v2

⇒ 16 = (4)(4) + (4)v2

⇒ 0 m/s = v2



Like Article
Suggest improvement
Previous
Center of Mass of Different Objects
Next
Linear Momentum of a System of Particles
Share your thoughts in the comments

Similar Reads

Difference between Center of Mass and Center of Gravity
The Total Mass of 1 mole electron is (mass of 1e-=9.1*10-28g)
Center of Mass of Different Objects
Write Examples of Uniform Motion and Non Uniform Motion
Difference between Rectilinear motion and Linear motion
Verification of the Law of Conservation of Mass in a Chemical Reaction
Formula Mass of Ionic Compounds
The Pressure Flow or Mass Flow Hypothesis
Growth Of Mass Nationalism
How to Calculate the Molar Mass of a Gas?

A
anjalishukla1859
Article Tags :
We use cookies to ensure you have the best browsing experience on our website. By using our site, you acknowledge that you have read and understood our Cookie Policy & Privacy Policy
Lightbox