Open In App

Proofs for the derivatives of eˣ and ln(x) – Advanced differentiation

Last Updated : 15 Dec, 2020
Improve
Improve
Like Article
Like
Save
Share
Report

In this article, we are going to cover the proofs of the derivative of the functions ln(x) and ex. Before proceeding there are two things that we need to revise:

The first principle of derivative

Finding the derivative of a function by computing this limit is known as differentiation from first principles. Derivative by the first principle refers to using algebra to find a general expression for the slope of a curve. It is also known as the delta method. The derivative is a measure of the instantaneous rate of change, which is equal to 

f^{\prime}(x)=\lim _{h \rightarrow 0} \frac{f(x+h)-f(x)}{h}

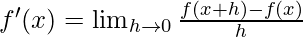
e in terms of limit

The number e, known as Euler’s number, is a mathematical constant approximately equal to 2.71828. The discovery of the constant itself is credited to Jacob Bernoulli in 1683 who attempted to find the value of the following expression (which is equal to e).

e=\lim _{n \rightarrow 0}(1+n)^{\frac{1}{n}}

Proof for the Derivative of ex

\begin{aligned} f^{\prime}(x)=& \lim _{h \rightarrow 0} \frac{f(x+h)-f(x)}{h} \\ =&\lim _{h \rightarrow 0} \frac{e^{x+h}-e^x}{h}\\ \qquad =&\lim _{h \rightarrow 0} \frac{e^{x}.e^h-e^x}{h} \\ \ \ =&e^x .\lim _{h \rightarrow 0} \frac{e^h-1}{h} \\ Let's \ take,\\ \qquad &e^{h}-1=n \\ we \ get,\\ \qquad &e^{h}=n+1 \\ \qquad &h=\ln (n+1) \\ &As\ \mathrm{h} \rightarrow 0 \Rightarrow n \rightarrow 0 \\ \qquad &=e^{x} \cdot \lim _{n \rightarrow 0} \frac{n}{\ln (n+1)} \\ \qquad &=e^{x} \cdot \lim _{n \rightarrow 0} \frac{1}{\frac{1}{n} \ln (n+1)} \\ \qquad &=e^{x} \cdot \lim _{n \rightarrow 0} \frac{1}{\ln (n+1)^{1 / n}} \\ \qquad &=e^{x} \cdot \frac{1}{\ln \lim _{n \rightarrow 0}(n+1)^{1 / n}} \\ \qquad\ &=e^{x} \cdot \frac{1}{\ln \mathrm{e}} \\ \qquad\ &=e^{x} \\ Hence, \\ \boldsymbol{{f}^{\prime}\left(e^{x}\right)=e^{x}} \end{aligned}

Example 1: Find the derivative of 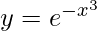y = {e^{ - {x^3}}}?

Solution:

By the chain rule,

{y^\prime = \left( {{e^{ - {x^3}}}} \right)^\prime }={ {e^{ - {x^3}}} \cdot \left( { - {x^3}} \right)^\prime }={ {e^{ - {x^3}}} \cdot \left( { - 3{x^2}} \right) }={ - 3{x^2}{e^{ - {x^3}}}.}

Example 2: Find the derivative of 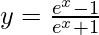y =\frac{{{e^x} - 1}}{{{e^x} + 1}}?

Solution:

Use here the quotient rule:

{y^\prime = \left( {\frac{{{e^x} - 1}}{{{e^x} + 1}}} \right)^\prime }={ \frac{{{e^x}\left( {{e^x} + 1} \right) - \left( {{e^x} - 1} \right){e^x}}}{{{{\left( {{e^x} + 1} \right)}^2}}} }={ \frac{{{\cancel{e^{2x}}} + {e^x} - \cancel{e^{2x}} + {e^x}}}{{{{\left( {{e^x} + 1} \right)}^2}}} }={ \frac{{2{e^x}}}{{{{\left( {{e^x} + 1} \right)}^2}}}.}

Proof for the Derivative of ln(x)

\begin{aligned} f^{\prime}(x)=& \lim _{h \rightarrow 0} \frac{f(x+h)-f(x)}{h} \quad\ \ \ \{\text { First Principle }\} \\ f^{\prime}(\ln x)=& \lim _{h \rightarrow 0} \frac{\ln (x+h)-\ln (x)}{h} \quad\{f(x+h)=\ln (x+h)\} \\ =& \lim _{h \rightarrow 0} \frac{\ln \left(\frac{x+h}{x}\right)}{h} \quad \quad\ \ \ \ \ \ \ \ \ \ \ \left\{\ln a-\ln b=\ln \frac{a}{b}\right\} \\ =& \lim _{h \rightarrow 0} \frac{\ln \left(1+\frac{h}{x}\right)}{h} \\ \text { Let's take, } \\ \qquad \frac{h}{x}=n \\ &A s h \rightarrow 0 \Rightarrow n \rightarrow 0\\ &=\lim _{n \rightarrow 0} \frac{\ln (n+1)}{n x}\\ &=\frac{1}{x} \cdot \lim _{n \rightarrow 0} \frac{\ln (n+1)}{n} \quad\{\text { taking } x \text { common }\}\\ &=\frac{1}{x} \cdot \lim _{n \rightarrow 0} \ln (n+1)^{1 / n} \quad\left\{a \cdot \log x=\log x^{a}\right\}\\ &=\frac{1}{x} \cdot \ln \lim _{n \rightarrow 0}(n+1)^{1 / n}\\ &=\frac{1}{x} \cdot \ln e \quad\{\text {From the defination of } e\}\\ &=\frac{1}{x} &\{\ln e=1\} \\ Hence, \\ f^{\prime}(\ln x)=\frac{1}{x} \end{aligned}

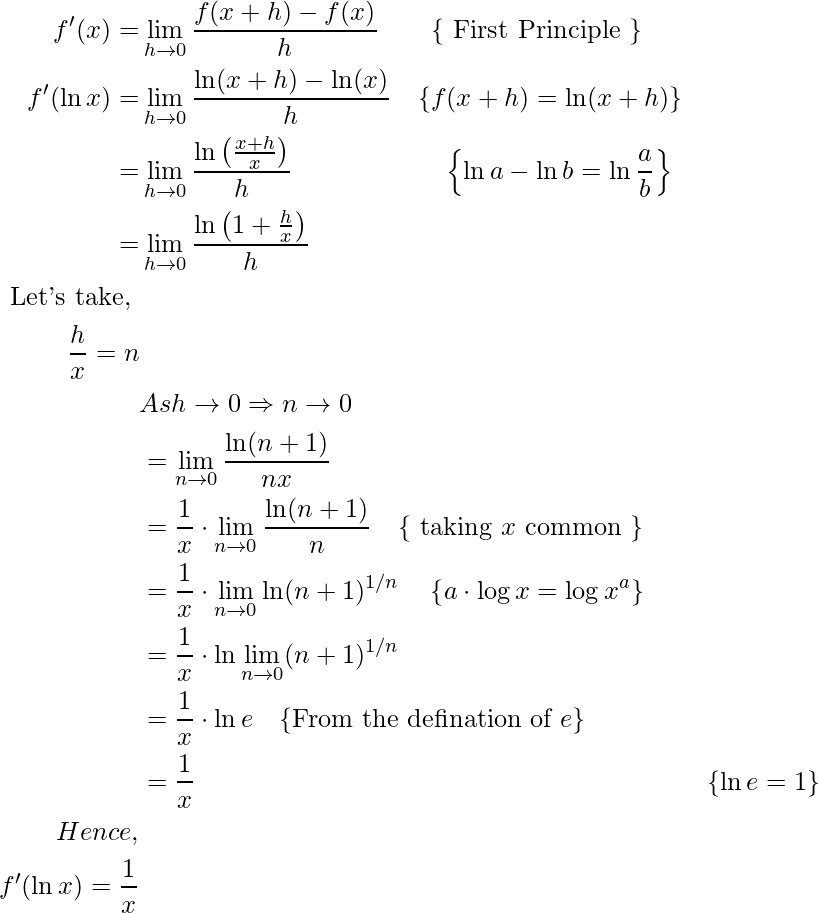
Example 1: Find the derivative of 3ln(x)?

Solution:

3ln(x)’ = 3(1/x) = 3/x

Example 2: Find the derivative of ln(x)/5?

Solution:

(ln(x)/5)’ = 1/5(ln(x))′ = (1/5) (1/x) = 1/5x



Like Article
Suggest improvement
Previous
Logarithmic Differentiation - Continuity and Differentiability
Next
Rolle's Theorem and Lagrange's Mean Value Theorem
Share your thoughts in the comments

Similar Reads

Disguised Derivatives - Advanced differentiation | Class 12 Maths
Implicit differentiation - Advanced Examples
Advanced Differentiation
De Morgan's Law - Theorem, Proofs, Formula & Examples
Logarithmic Differentiation - Continuity and Differentiability
Differentiation, Dedifferentiation and Redifferentiation in Plant Growth
Differentiation and Integration Formula
Logarithmic Differentiation
Class 12 RD Sharma Solutions - Chapter 11 Differentiation - Exercise 11.6
Class 12 RD Sharma Solutions- Chapter 11 Differentiation - Exercise 11.8 | Set 1

R
rathoreatul27
Article Tags :
We use cookies to ensure you have the best browsing experience on our website. By using our site, you acknowledge that you have read and understood our Cookie Policy & Privacy Policy
Lightbox